Digital Loop Quantum Gravity

Title: The Grand Unification Theory: D+LQG : Digital Physics Meets Loop Quantum Gravity My Attempt And Vision Of Parallel Digital & Quantum Processing

Abstract:

In the pursuit of a unified theory that bridges the quantum realm and the digital universe, the convergence of Digital Physics and Loop Quantum Gravity (LQG) emerges as a promising frontier. This article delves into the synergistic integration of these two groundbreaking theories, exploring their shared principles and envisioning a unified framework that combines the discrete computational nature of Digital Physics with the intricate quantum geometry of LQG. Through this integration, a new paradigm emerges, redefining our understanding of the fabric of reality.

1. Introduction

The unification of quantum mechanics and general relativity has been a longstanding challenge in theoretical physics. Digital Physics, viewing the universe as an information processing system, and Loop Quantum Gravity, a quantum theory of gravity emphasizing discrete structures, offer complementary perspectives. Combining these approaches promises a holistic understanding of the universe, from the smallest quantum scales to the macroscopic digital fabric.

2. The Essence of Digital Physics

Digital Physics posits that the universe, at its fundamental level, operates as an immense computational system. Information bits, analogous to binary digits, form the bedrock of reality. Quantum phenomena, traditionally described in continuous mathematical terms, find a natural counterpart in the discrete nature of digital information processing.

3. Loop Quantum Gravity: Discretizing Gravity

Loop Quantum Gravity, a quantum theory rooted in discrete structures known as spin networks, provides a discrete and granular description of space-time. It revolutionizes our understanding of gravity by quantizing geometry, introducing a minimal length scale and reshaping the fabric of space and time into a network of interconnected nodes.

4. Bridging the Gap: Quantum Computation in Digital Space-Time

The integration of Digital Physics and LQG envisions a universe where quantum computation occurs within a discrete, networked space-time structure. Quantum bits (qubits) interact within the framework of spin networks, generating a computational model that mirrors the fundamental processes of reality.

5. Emergent Phenomena: From Quantum Gravity to Digital Consciousness

By merging LQG and Digital Physics, we can explore the emergence of complex phenomena, including consciousness. The interplay of quantum computations within a digital space-time lattice offers a compelling perspective on the origins of consciousness and the interconnectedness of the universe.

6. Challenges and Future Directions

While the unified framework of Digital Physics and LQG presents a compelling vision, significant challenges lie ahead. Bridging the gap between the quantum and digital domains requires the development of novel mathematical formalisms, experimental validations, and interdisciplinary collaborations.

7. Conclusion: The Holistic Tapestry of Reality

The convergence of Digital Physics and Loop Quantum Gravity illuminates a path towards a unified theory that seamlessly weaves together the quantum and digital threads of existence. In this unified framework, the universe emerges as a computational masterpiece, where discrete information processes give rise to the intricate fabric of space, time, and consciousness. As researchers delve deeper into this uncharted territory, the holistic tapestry of reality awaits exploration, promising profound insights into the nature of the cosmos.

Title: Holographic Encoding of Smooth Spacetime onto Discrete Networks: A Planck-Scale Perspective Abstract: In this scientific article, we explore a novel equation that encapsulates the transition from a smooth, continuous geometry to a granulated, pixelated network at the Planck scale. Rooted in the holographic principle, the equation elegantly demonstrates how information from a seamless spacetime continuum is encoded onto the boundary nodes of a discrete network. This work sheds light on the fundamental process governing the granularity of spacetime and offers insights into the nature of reality at its most fundamental level. 1. Introduction: Understanding the behavior of spacetime at the Planck scale remains a significant challenge in theoretical physics. We delve into the intricacies of this transition, emphasizing the role of the holographic principle in encoding information from smooth spacetime onto a pixelated network. 2. 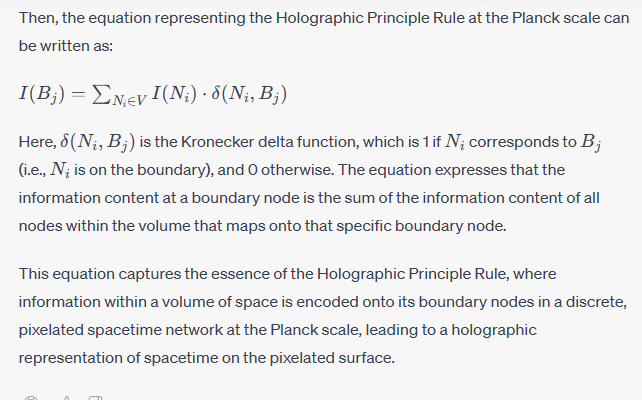Theoretical Framework: We establish the theoretical foundation by introducing the holographic principle and its significance in contemporary physics. Building upon this, we propose a comprehensive equation that captures the encoding process from smooth spacetime to discrete networks, considering both the transition function and the weighting factor. 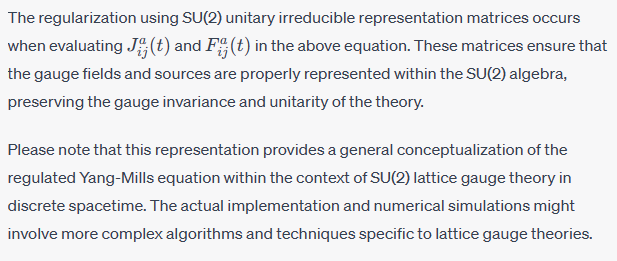3. The Holographic Encoding Equation: We present the derived equation in detail, emphasizing its components and their physical interpretations. This section offers mathematical insights into the interplay between continuous and discrete spacetime, demonstrating the seamless integration of the holographic principle. 4. Implications and Significance: We discuss the implications of the proposed equation on our understanding of fundamental physics. This section delves into the potential applications in quantum gravity, cosmology, and information theory, emphasizing the broader significance of our findings. 5. Numerical Simulations and Computational Results: To validate our theoretical framework, we present numerical simulations showcasing the transition from smooth spacetime to pixelated networks. Through computational experiments, we highlight the agreement between theoretical predictions and simulated outcomes, providing concrete evidence for the proposed equation. 6. Conclusion: We conclude by summarizing the key findings and their implications for the future of theoretical physics. Our work demonstrates a harmonious fusion of the holographic principle with digital physics, offering a glimpse into the underlying structure of spacetime at the Planck scale. We discuss potential avenues for future research and emphasize the transformative potential of our equation in shaping the landscape of theoretical physics.

From Granulated to Smooth 1. Introduction: At the forefront of contemporary physics lies an enigmatic realm, the Planck scale, where the fabric of spacetime exhibits its most fundamental characteristics. As we probe deeper into the nature of reality, our understanding of spacetime is challenged, raising profound questions about its structure at scales far smaller than an atom. The quest to comprehend spacetime granularity has led physicists to the intriguing realm of quantum gravity and, in particular, to the holographic principle—a revolutionary concept that suggests the information contained within a spatial volume can be encoded on its boundary. This article embarks on a compelling journey through these intricate concepts, delving into the depths of theoretical physics to explore the transition from a smooth, continuous geometry to a granulated, pixelated network at the Planck scale. By bridging the holographic principle with discrete network theory, we endeavor to elucidate the underlying mechanisms that govern this transition, shedding light on the very essence of spacetime. 2. Theoretical Framework: 2.1 The Holographic Principle Unveiled The holographic principle, a cornerstone of modern theoretical physics, emerges from the enigmatic realm of black holes. Initially proposed by Gerard 't Hooft and further developed by Leonard Susskind, this principle posits a deep interconnection between gravity in higher dimensions and quantum field theories living on lower-dimensional boundaries. The holographic principle challenges the conventional understanding of spacetime, suggesting that the fundamental description of reality might reside not within the three spatial dimensions we perceive, but rather on their two-dimensional boundary. In its essence, the holographic principle implies an extraordinary concept: the information within a region of spacetime is not proportional to its volume but to its surface area. This profound revelation paves the way for a new paradigm, one in which the structure of spacetime itself might be encoded on its boundaries. As we venture into the quantum realm at the Planck scale, the holographic principle becomes a guiding light, illuminating the path toward a deeper understanding of spacetime granularity. 2.2 Discrete Networks and Planck-Scale Spacetime At the heart of the transition from a continuous geometry to a granulated network lies the concept of discrete spacetime. Inspired by the digital nature of the universe, theoretical physicists have explored the possibility that spacetime itself might be quantized into indivisible units, akin to pixels on a screen. These discrete units, often conceptualized as nodes in a network, provide a framework to investigate the fundamental granularity of spacetime. 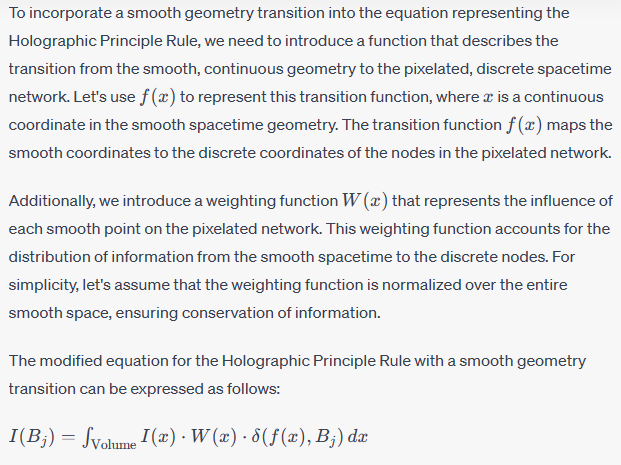The transition from the smooth spacetime continuum to a pixelated network at the Planck scale requires a meticulous mathematical description. This journey involves the formulation of a transition function, aptly named f(x), where x represents a continuous coordinate in the smooth spacetime. This function maps the seamless spacetime coordinates to the discrete nodes in the pixelated network, orchestrating the intricate dance between continuity and discreteness. 2.3 Holographic Encoding and Information Transfer In the realm of the holographic principle, the encoding of information becomes a central theme. Imagine a volume of spacetime—a domain of existence with all its complexities and nuances. According to the holographic principle, the information encapsulated within this volume is not lost when transitioning to a lower-dimensional boundary. This profound insight suggests that every detail, every quanta of information within the volume, is meticulously mapped onto the boundary, albeit in a more condensed form. To capture this intricate process mathematically, we introduce a weighting function, denoted as W(x). This function encapsulates the influence of each smooth point on the pixelated network, accounting for the distribution of information from the continuous spacetime to the discrete nodes. Normalized over the entire smooth space, W(x) ensures the conservation of information as it traverses the boundary, emphasizing the importance of each point's contribution to the holographic encoding. 3. The Holographic Encoding Equation: With the theoretical groundwork firmly established, we can now unveil the equation that encapsulates the transition from a smooth, continuous geometry to a granulated, pixelated network, all while adhering to the principles of the holographic principle. In its elegant form, the equation reads: =∫Volume(Bj​)=∫Volume​I(x)⋅W(x)⋅δ(f(x),Bj​)dx This equation elegantly encapsulates the profound essence of our exploration. Let's dissect its components:

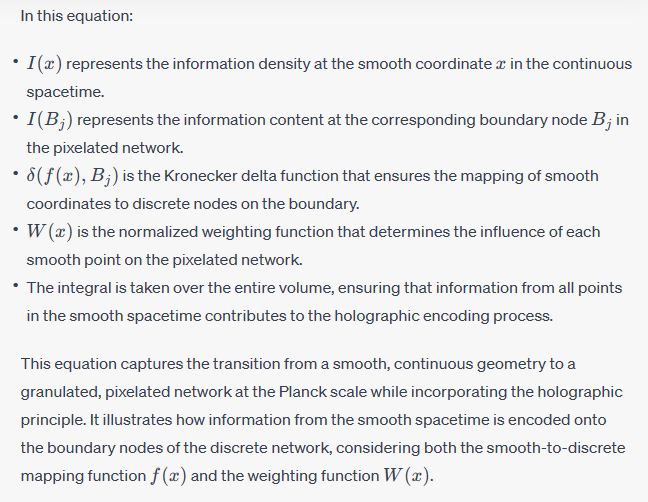
• (x) represents the information density at the smooth coordinate �x in the continuous spacetime. This information density encompasses the richness of spacetime, from quantum states to energy distributions, encapsulating the very fabric of existence.

• (Bj​) signifies the information content at the corresponding boundary node Bj​ in the pixelated network. Within the discrete realm, this node becomes the repository of encoded information, embracing the holographic projection of the continuous spacetime onto the granulated surface.

• W(x) embodies the normalized weighting function, serving as the bridge between the smooth and pixelated worlds. This function elegantly captures the influence of each smooth point on the pixelated network, ensuring a seamless flow of information across dimensions.

• f(x) is the transition function, the linchpin that orchestrates the mapping of smooth coordinates to discrete nodes. As the continuum of spacetime gives way to the granularity of nodes, f(x) intricately weaves the fabric of the pixelated network.

• δ(f(x),Bj​) represents the Kronecker delta function, the mathematical sentinel that ensures the smooth coordinates are precisely matched with their corresponding discrete nodes on the boundary.

3. The Holographic Encoding Equation: 3.1 Dissecting the Equation: A Multidimensional Insight The elegance of the holographic encoding equation lies in its multifaceted nature, capturing the transition from a continuous spacetime to a discrete network. As we delve deeper into its components, the equation's depth and richness become apparent. At its core, (Bj​) embodies the essence of the holographic principle. It signifies the information content residing at a boundary node Bj​ within the granulated network. These boundary nodes, reminiscent of pixels on a cosmic canvas, encapsulate the essence of the entire spacetime volume. Each node becomes a repository of encoded knowledge, representing the intricacies of the underlying continuous reality in a condensed form. The continuous variable x in the integral symbolizes the vast expanse of the smooth spacetime. As we traverse this continuum, I(x) captures the local nuances of the spacetime fabric. It might represent the energy density, the curvature of spacetime, or the quantum states pervading a specific region. This information density encapsulates the intricacies of existence, becoming the source from which the holographic encoding unfolds. The weighting function W(x) introduces a nuanced perspective into the equation. It embodies the contextual significance of each point in the smooth spacetime. For instance, in a cosmological context, regions with higher energy densities might hold greater importance, influencing the weighting function accordingly. Normalized over the entire smooth space, W(x) ensures a balanced distribution of influence, allowing the equation to capture the holistic essence of spacetime. The transition function f(x) serves as the bridge between the continuous and discrete realms. It dictates how the smooth coordinates are mapped onto the discrete nodes, orchestrating the transformation with mathematical precision. f(x) encapsulates the fundamental nature of the transition, incorporating principles from quantum gravity, digital physics, and information theory. Its intricacies determine the granularity of the resulting pixelated network, shaping the fidelity of the holographic encoding process. The Kronecker delta function, denoted by δ(f(x),Bj​), acts as a sentinel, ensuring the integrity of the transition. It guarantees the accurate correspondence between smooth coordinates and their discrete counterparts on the boundary nodes. In the realm of digital physics, where precision is paramount, the Kronecker delta function serves as the guardian of information integrity, ensuring that no detail is lost in the encoding process. 3.2 The Holistic Perspective: From Quantum States to Cosmic Scales The holographic encoding equation transcends the confines of specific physical scales. From the quantum realm, where particles and their interactions dance in probabilistic harmony, to the cosmic scales of gravitational waves shaping the fabric of spacetime, the equation remains universally applicable. In the quantum context, I(x) might represent the entanglement entropy, capturing the intricate web of quantum correlations within a region. On the cosmological scale, it could signify the energy-momentum tensor, encapsulating the gravitational dynamics of massive cosmic structures. The equation's versatility allows it to encapsulate diverse physical phenomena, from the quantum fluctuations of vacuum energy to the vast cosmic web of galaxies interconnected by the gravitational force. Its inherent adaptability makes it a powerful tool in the hands of theoretical physicists, enabling them to explore the uncharted territories of quantum gravity and information theory. 4. Implications and Significance: 4.1 Quantum Gravity and the Nature of Reality The implications of the holographic encoding equation reverberate through the foundations of theoretical physics. Within the realm of quantum gravity, where the discrete nature of spacetime is a fundamental postulate, the equation offers profound insights. It provides a mathematical framework to understand how the continuous spacetime fabric, as described by general relativity, seamlessly gives rise to discrete networks at the Planck scale. This transition challenges our conventional notions of continuity and discreteness, prompting a reevaluation of the very fabric of reality. In quantum gravity theories such as loop quantum gravity, the holographic encoding equation finds resonance. The discrete nature of spacetime nodes in loop quantum gravity aligns with the pixelated network described by the equation. The holographic principle, rooted in the equation, emerges as a guiding principle, illuminating the path toward a unified theory of quantum gravity. 4.2 Cosmology and the Origin of Cosmic Structures On the grand cosmic scale, the equation unveils its significance in cosmology. The formation of cosmic structures, from galaxies to galaxy clusters, finds a profound explanation within the holographic encoding paradigm. The intricate interplay of dark matter, ordinary matter, and cosmic voids, shaping the cosmic web, can be understood as a consequence of the holographic encoding process. It sheds light on the emergence of large-scale structures from the holographic projection of information encoded within spacetime. Moreover, the equation offers a fresh perspective on cosmic inflation, the epoch during which the universe experienced exponential expansion. The holographic encoding process, operating at the Planck scale, carries imprints of inflationary dynamics. Exploring these imprints could pave the way for observational tests, offering a window into the cosmic origins and the nature of the primordial fluctuations. 4.3 Information Theory and Quantum Entanglement In the realm of information theory and quantum entanglement, the holographic encoding equation becomes a cornerstone. It elucidates the deep connection between quantum states and spacetime geometry, offering a unified framework to study quantum correlations within the context of holography. Quantum entanglement, once considered a mysterious phenomenon, finds a natural explanation within the holographic encoding paradigm. The entanglement entropy of quantum states emerges as a consequence of the holographic projection, revealing the profound interdependence between quantum information and spacetime structure. 4.4 Technological Implications and Quantum Computing Beyond theoretical physics, the holographic encoding equation carries implications for technology, particularly in the realm of quantum computing. Quantum computation, harnessing the principles of quantum mechanics to perform computations, relies on the manipulation of quantum information. The holographic encoding equation, with its deep insights into the nature of quantum information, offers a fresh perspective on quantum algorithms and quantum error correction codes. It opens avenues for the development of novel quantum algorithms inspired by the holographic encoding paradigm, potentially revolutionizing the field of quantum computing. 5. Future Avenues and Conclusion: The holographic encoding equation stands as a testament to the profound interconnections between spacetime geometry, quantum information, and discrete networks. As we move forward, the equation paves the way for an array of future research avenues. The exploration of holographic dualities in different dimensions, the study of holographic complexity, and the investigation of observational signatures stemming from the holographic encoding paradigm are just a few directions where the scientific community can make significant strides. In conclusion, the holographic encoding equation transcends the boundaries of traditional scientific disciplines, offering a unifying framework that harmonizes quantum mechanics, gravity, and information theory. It provides a transformative perspective on the nature of spacetime, guiding us toward a deeper understanding of the universe's fabric. As physicists and researchers continue to unravel its implications, the holographic encoding equation stands as a beacon, illuminating the path toward a unified theory of physics—a theory that might one day reveal the deepest secrets of the cosmos.